top button
Flag Notify
    Connect to us
      Site Registration

Site Registration

Connecting the midpoints of a quadrilateral's four sides forms a square. Must the original quadrilateral be a square?

0 votes
256 views
Connecting the midpoints of a quadrilateral's four sides forms a square. Must the original quadrilateral be a square?
posted Sep 24, 2018 by anonymous

Share this puzzle
Facebook Share Button Twitter Share Button LinkedIn Share Button

1 Answer (Check Answer ▼)





Similar Puzzles
0 votes
A person makes cuts linking the midpoints of every 2 adjacent sides and distributes these 6 slices to his friends....

A Greedy person is celebrating his birthday with 6 of his friends. His mother baked him a birthday cake in the shape of a regular hexagon. Wanting to keep most of the cake, he makes cuts linking the midpoints of every 2 adjacent sides, and distributes these 6 slices to his friends. What proportion of the cake does he have left for himself?

enter image description here

0 votes
Choose the best word for the blank: If a quadrilateral with two pairs of consecutive congruent sides...

Let ABC be an acute triangle with angle A = 60 and let D be the midpoint of BC. The points E and F are the feet of the altitudes from B and C respectively. Which one of the given descriptions best describe triangle DEF?

enter image description here

a) Right angled
b) Isoceles
c) Equilateral
d) Scalene

+1 vote
9 unit circles are packed into a square. What is the length of longest path connecting two opposite corners of square?

Nine unit circles are packed into a square, tangent to their neighbors and to the square. What is the length of the longest smooth path connecting two opposite corners of the square?

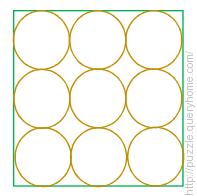
nine squares

Assumptions:
- The path must be continuous and follow the lines in the diagram; that is, it must be made up of portions of either the circles or the outside square.
- The path may not change direction suddenly.
- The path may not contain any loops.
- The path may not touch or cross itself at any point.

0 votes
A cuboid with sides in the ratio of 8:5:6 is cut perpendicular...........what is the volume of original cuboid (in cm2)?

A cuboid with sides in the ratio of 8:5:6 is cut perpendicular its length to form three smaller cuboids of equal sides.
If sum of the surface areas of all three cuboids is 1080 cm2 is more than the surface area of original cuboid, then what is the volume of original cuboid (in cm2)?

...