top button
Flag Notify
    Connect to us
      Site Registration

Site Registration

A semicircle contains an inscribed semicircle, as shown, dividing its diameter into lengths of 2 and 1.............

0 votes
273 views

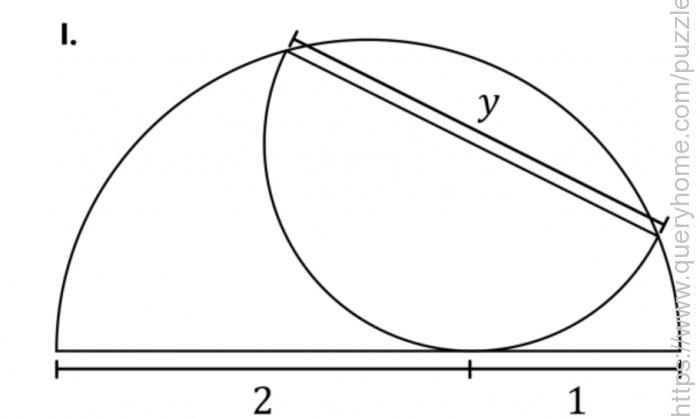
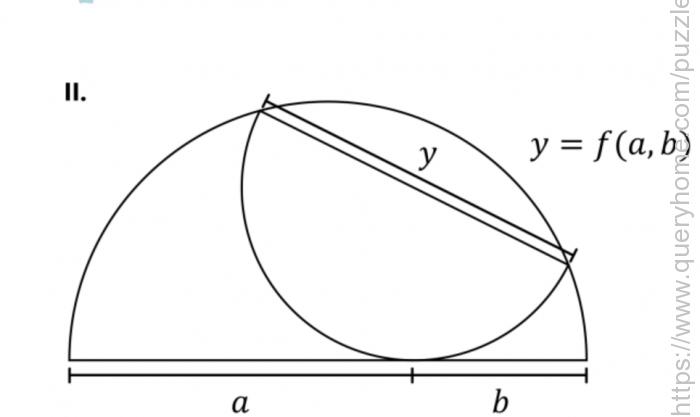
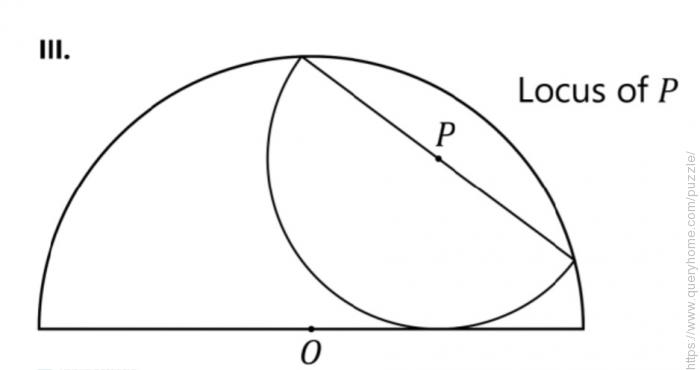
A semicircle contains an inscribed semicircle, as shown, dividing its diameter into lengths of 2 and 1. The question has three parts.

I. What is the value y equal to the length of the inscribed semicircle’s diameter?

II. Solve again for the general case if the large diameter is divided into lengths of a and b.

III. Let P be the center of the inscribed semicircle. Solve for the locus of P–that is the set of points P for all possible inscribed semicircles.
enter image description here

enter image description here

enter image description here

posted Feb 11, 2022 by Vikram Luthra

Looking for solution? Promote on:
Facebook Share Button Twitter Share Button LinkedIn Share Button




Similar Puzzles
0 votes
A circle of radius 12 is inscribed in a right triangle, dividing the hypotenuse into lengths of a and b = 44............

A circle of radius 12 is inscribed in a right triangle, dividing the hypotenuse into lengths of a and b = 44.
What is the length of a?
enter image description here

+1 vote
A semicircle with radius a is inscribed in a rectangle with its diameter along one...........Solve for the value of a/b.

A semicircle with radius a is inscribed in a rectangle with its diameter along one side of the rectangle. A circle with radius b is then inscribed so it is tangent to the rectangle and the semicircle, as shown.
Solve for the value of a/b.

enter image description here

+2 votes
A square is inscribed along the right angle of a right triangle, as shown, with vertical and horizontal lengths.........

A square is inscribed along the right angle of a right triangle, as shown, with vertical and horizontal lengths to the corners of 5 and 20. What is the area of the square?
enter image description here

0 votes
A semicircle is inscribed in a right triangle as shown. What is the area of the shaded region in the figure?

A semicircle is inscribed in a right triangle as shown. What is the area of the shaded region in the figure?
enter image description here

0 votes
A square contains a semicircle and a quarter circle, as shown. The two circles are tangent to each other................

A square contains a semicircle and a quarter circle, as shown. The two circles are tangent to each other. The semicircle has a diameter equal to 12 and the quarter circle has a radius equal to 12.
What is the area of the square?
What is the length of the tangent line segment AB?

enter image description here

...