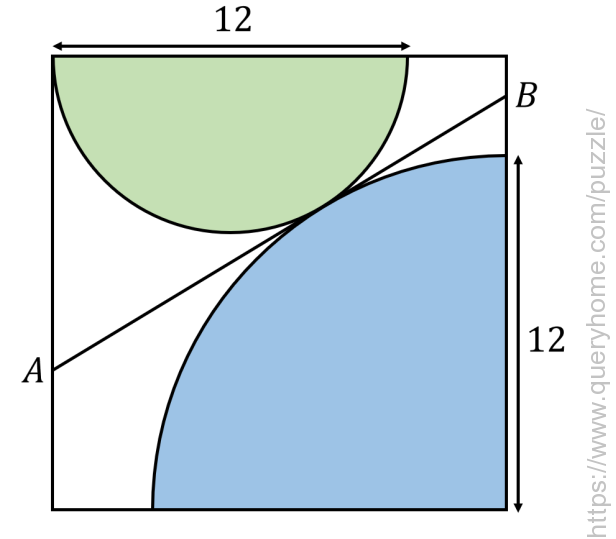
A square contains a semicircle and a quarter circle, as shown. The two circles are tangent to each other. The semicircle has a diameter equal to 12 and the quarter circle has a radius equal to 12.
What is the area of the square?
What is the length of the tangent line segment AB?